On November 19, 2017, the Mathematical Sciences Research Institute (MSRI) held a private event in Sonoma County for members of the Museion Society in partnership with Llisa Eames Demetrios, granddaughter of renowned designers Charles and Ray Eames, and her husband Mark Burstein, a lifelong, second-generation collector, president emeritus of the Lewis Carroll Society of North America, and editor or introducer of, or contributor to fourteen books about Carroll, including The Annotated Alice: 150th Anniversary Deluxe Edition (W. W. Norton, 2015) and Alice’s Adventures in Wonderland: 150th Anniversary Edition Illustrated by Salvador Dalí (Princeton University Press, 2015).
On November 19, 2017, the Mathematical Sciences Research Institute (MSRI) held a private event in Sonoma County for members of the Museion Society in partnership with Llisa Eames Demetrios, granddaughter of renowned designers Charles and Ray Eames, and her husband Mark Burstein, a lifelong, second-generation collector, president emeritus of the Lewis Carroll Society of North America, and editor or introducer of, or contributor to fourteen books about Carroll, including The Annotated Alice: 150th Anniversary Deluxe Edition (W. W. Norton, 2015) and Alice’s Adventures in Wonderland: 150th Anniversary Edition Illustrated by Salvador Dalí (Princeton University Press, 2015).
Friend of MSRI and Math Lovers Forum sponsor Ashok Vaish was in attendance at the event and shares the following reflections on the experience on his personal blog, reposted below.
Last Sunday (November 19, 2017) we entered through the beautiful blue steel gates of the Eames house in Sonoma County and were immediately transformed into the magic of Charles and Ray Eames’ world of art, function, mathematics and whimsy. We were hosted by Llisa Eames Demetrios, Eames’ granddaughter, who herself is an artist and an accomplished sculptor, and her husband, Mark Burstein, who has a fantastic collection of Alice in Wonderland memorabilia. He has a tower full of books — tens of thousands of them — and other fun objects all related to capturing the imaginative writings, the mathematical undertones and the dreamlike artwork associated with Lewis Clark’s classic. One fascinating folio contained artwork by Salvador Dali to annotate Alice in Wonderland.

Seen in this picture from Mark’s folio (which he doesn’t usually open for visitors because it is fragile and precious) is Dali’s beautiful illustration, alongside a California license plate about the Mock Turtle. Note the text printed in the form of a mouse’s tail and Dali’s swishy-tailed mouse in the picture! There are dozens of such stunningly beautiful Dali paintings in this book. Mark is full of passion for all things delightfully whimsical — he recited “nonsense” poetry by Ogden Nash and delighted in mathematical curiosities and lateral thinking — jumping from a set of ideas from one realm of learning to another seemingly unrelated one. His is a world of puzzles, adventures, Martin Gardner, surrealism and imaginative poetry!
We went there at the invitation of MSRI, the Mathematical Sciences Research Institute in Berkeley, which promotes this kind of wonderful thinking. Their Museion Society membership is a great way to join their outreach and get invited to such functions. Llisa and Mark laid out a sumptuous lunch, gave us a leisurely tour of their premises and even showed us a nice video interview of Charles Eames, which they projected on the ceiling while we lay on cushions! All in all a treat for the mind and the senses.
I want to highlight one piece of beautiful artwork bringing together two fascinating mathematical curiosities.
1. Knight’s Tour

The painting above shows a knight’s tour. What is a knight’s tour? It is a journey by a knight across the chessboard starting at a given point and traversing all 64 squares while visiting every square just once. Knights tours are a big mathematical curiosity and have only been partially understood, even today with modern computers. For a 8 by 8 chessboard trillions of knight’s tours are possible. Knight’s tours can also form a closed loop — topologically a circle route through the 64 squares of a chessboard. There are more than 26 trillion possible closed tours!
Here’s an excerpt from the Wikipedia:
On an 8 × 8 board, there are exactly 26,534,728,821,064 directed closed tours (i.e. two tours along the same path that travel in opposite directions are counted separately, as are rotations and reflections). The number of undirected closed tours is half this number, since every tour can be traced in reverse. There are 9,862 undirected closed tours on a 6 × 6 board.
It has only been possible to calculate these numbers recently thanks to great algorithms developed by the likes of the legendary Don Knuth. So you can see that the knight’s tour is a fascinating problem. Incidentally there are no closed loops possible on an odd numbered chessboard e. g. a board with 7 squares to a side. Can you see why?
The ancient Hindus, who invented the earliest form of chess, were fascinated by the knight’s tour problem. In the 9th century AD a Sanskrit poem by Rudrata presents the knight’s tour as an elaborate metric composition, the “city-alankara”.
2. Magic Squares
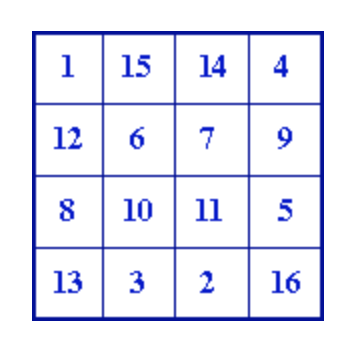
The second mathematical curiosity is the magic square. A magic square is an arrangement of the numbers from 1 to n-squared in an nxn matrix, with each number occurring exactly once, and such that the sum of the entries of any row, any column, or any main diagonal is the same. In the figure to the right we have 4X4 magic square with numbers from 1 to 16 arranged in a 4X4 pattern and each row, column and diagonal adding up to 34.
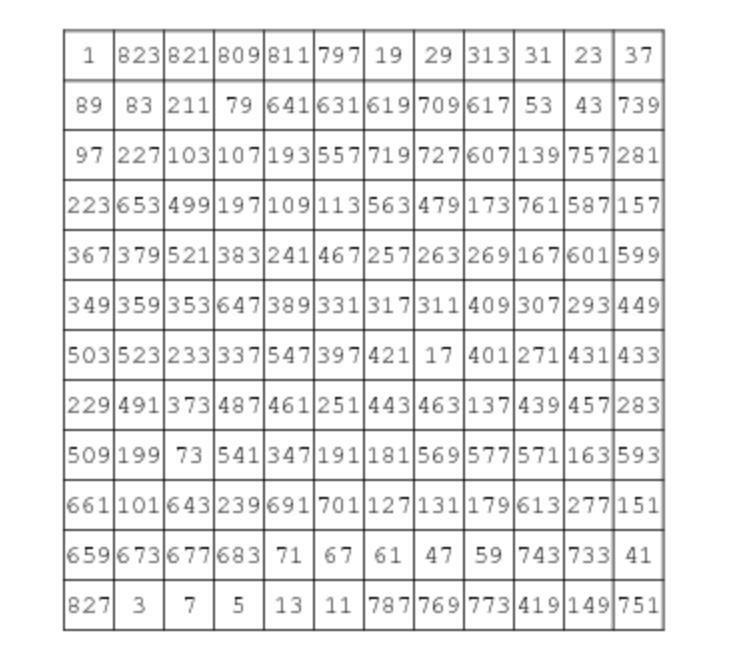
Magic squares, like knight’s tours, occur with great frequencies and form beautiful objects. It is possible to build a magic square of virtually every square size. Astonishingly they can also be made from such rarities as consecutive primes instead of consecutive numbers. Prime number magic squares can be made of almost any square size, but consecutive primes starting with 1 is another matter. (1 is technically not a prime but is sometimes allowed for prime magic squares). Here is the smallest magic square with 144 consecutive primes starting with 1 (size 12X12). Only odd primes are used, it’s hard to include 2. (The Example is taken from Wikipedia, of course.)
3. Combining Knight’s Tour and Magic Squares
Putting these two great mathematical curiosities together we come back to the painting at the Eames’ home of the knight’s tour. Not only is it a geometrically pleasing traversal of the board by a knight, it is also a magic square! All rows, columns and diagonals add up to 260. Check it out.
Fascinating! This was one of many engrossing items at the Eames’ home and I feel lucky to have had a chance to sip from their magic fountain.
Originally published at The Capitalist Muse.